Unit 5 Polynomial Functions Homework 1 embarks on a comprehensive exploration of polynomial functions, unveiling their multifaceted nature and practical applications. This homework assignment serves as a cornerstone for understanding the behavior, properties, and diverse uses of these essential mathematical tools.

Polynomial functions, characterized by their non-negative integer exponents, exhibit a wide range of properties that govern their continuity, differentiability, and other attributes. Homework 1 delves into these properties, providing a solid foundation for further exploration.

Polynomial Functions: Unit 5 Polynomial Functions Homework 1

Polynomial functions are a fundamental class of functions that play a vital role in various mathematical applications. They are defined as functions that can be expressed as the sum of terms, where each term consists of a constant multiplied by a non-negative integer power of a variable.

The degree of a polynomial function is the highest power of the variable that appears in the function.

Examples of polynomial functions include:

• Linear function: f(x) = ax + b
• Quadratic function: f(x) = ax ²+ bx + c
• Cubic function: f(x) = ax ³+ bx ²+ cx + d

Polynomial functions possess several important properties, including:

• Continuity: Polynomial functions are continuous at all points in their domain.
• Differentiability: Polynomial functions are differentiable at all points in their domain.
• Integrability: Polynomial functions can be integrated using standard techniques.

Homework 1

Homework 1 provides an opportunity to practice the concepts of polynomial functions covered in class. It includes problems that require students to:

• Evaluate polynomial functions at given values of the variable.
• Factor polynomial functions.
• Graph polynomial functions.
• Solve equations involving polynomial functions.

To successfully solve these problems, students should:

• Understand the concepts of polynomial functions and their properties.
• Be familiar with the various methods of factoring polynomial functions.
• Have a strong foundation in algebra and calculus.

Applications of Polynomial Functions

Polynomial functions have numerous applications in various fields, including:

• Physics:Polynomial functions can be used to model the trajectory of projectiles, the motion of objects in a gravitational field, and the vibrations of objects.
• Engineering:Polynomial functions are used in the design of bridges, buildings, and other structures.
• Economics:Polynomial functions can be used to model supply and demand, consumer behavior, and economic growth.

For example, the polynomial function f(x) = -0.004x ³+ 0.12x ²– 1.44x + 5.76 can be used to model the average monthly temperature in degrees Celsius for a given month x in a particular city.

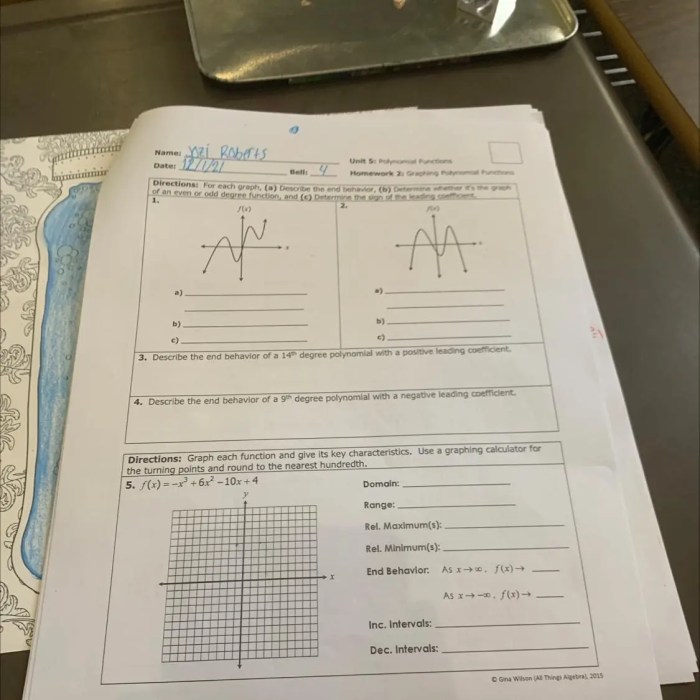
Polynomial Function Graphs, Unit 5 polynomial functions homework 1

Graphing polynomial functions involves finding the key features of the graph, such as the intercepts, the vertex, and the end behavior. The graph of a polynomial function can be determined by:

• Finding the intercepts by setting y = 0 and solving for x.
• Finding the vertex by using the formula x = -b/2a.
• Determining the end behavior by looking at the degree of the polynomial function.

For example, the graph of the polynomial function f(x) = x ²– 4 has intercepts at (0, -4) and (0, 4), a vertex at (0, -4), and end behavior that increases without bound as x approaches infinity and decreases without bound as x approaches negative infinity.

Polynomial Function Transformations

Polynomial function transformations involve applying operations to the graph of a polynomial function to create a new graph. These transformations include:

• Translations:Moving the graph up, down, left, or right.
• Reflections:Flipping the graph over the x-axis or y-axis.
• Stretches:Vertically or horizontally stretching the graph.

For example, the graph of the polynomial function f(x) = (x – 2) ²is a translation of the graph of f(x) = x ²by 2 units to the right.

Clarifying Questions

What are the key concepts covered in Unit 5 Polynomial Functions Homework 1?

Homework 1 focuses on the definition, properties, and applications of polynomial functions, including their continuity, differentiability, and graphing techniques.

What types of problems are included in Homework 1?

Homework 1 encompasses a variety of problems, such as simplifying polynomial expressions, finding roots and zeros, graphing polynomial functions, and applying polynomial functions to real-world scenarios.

How can I improve my problem-solving skills for polynomial functions?

Practice is key! Engage in regular problem-solving exercises, analyze different types of problems, and seek guidance from instructors or peers to enhance your understanding.